What is the absolute value of a function?
The absolute value of a function is a measure of the distance between the function's output and zero. It is defined as the output of the function if the output is positive, or the negative of the output if the output is negative. For example, the absolute value of the function f(x) = x is |x|, which is equal to x if x is positive and -x if x is negative.
The absolute value of a function has a number of important properties. First, it is always non-negative. Second, it is symmetric about the y-axis. Third, it is a continuous function. These properties make the absolute value of a function useful for a variety of applications, including:
- Finding the distance between two points
- Measuring the error in a calculation
- Solving equations and inequalities
The absolute value of a function is a fundamental concept in mathematics. It has a wide range of applications and is an essential tool for understanding many different types of problems.
Absolute Value of a Function
The absolute value of a function is a fundamental concept in mathematics. It has a wide range of applications and is an essential tool for understanding many different types of problems.
- Definition: The absolute value of a function is the distance between the function's output and zero.
- Properties: The absolute value of a function is always non-negative, symmetric about the y-axis, and continuous.
- Applications: The absolute value of a function can be used to find the distance between two points, measure the error in a calculation, and solve equations and inequalities.
- Historical Context: The concept of the absolute value of a function was first developed by Ren Descartes in the 17th century.
- Related Concepts: The absolute value of a function is related to the concepts of modulus and distance.
- Examples: The absolute value of the function f(x) = x is |x|, which is equal to x if x is positive and -x if x is negative.
- Importance: The absolute value of a function is a fundamental tool for understanding many different types of mathematical problems.
These are just a few of the key aspects of the absolute value of a function. This concept is a powerful tool that has a wide range of applications in mathematics and beyond.
Definition
This definition is important because it provides a clear and concise way to understand what the absolute value of a function is. It also highlights the fact that the absolute value of a function is always non-negative. This is because the distance between any two points is always non-negative.
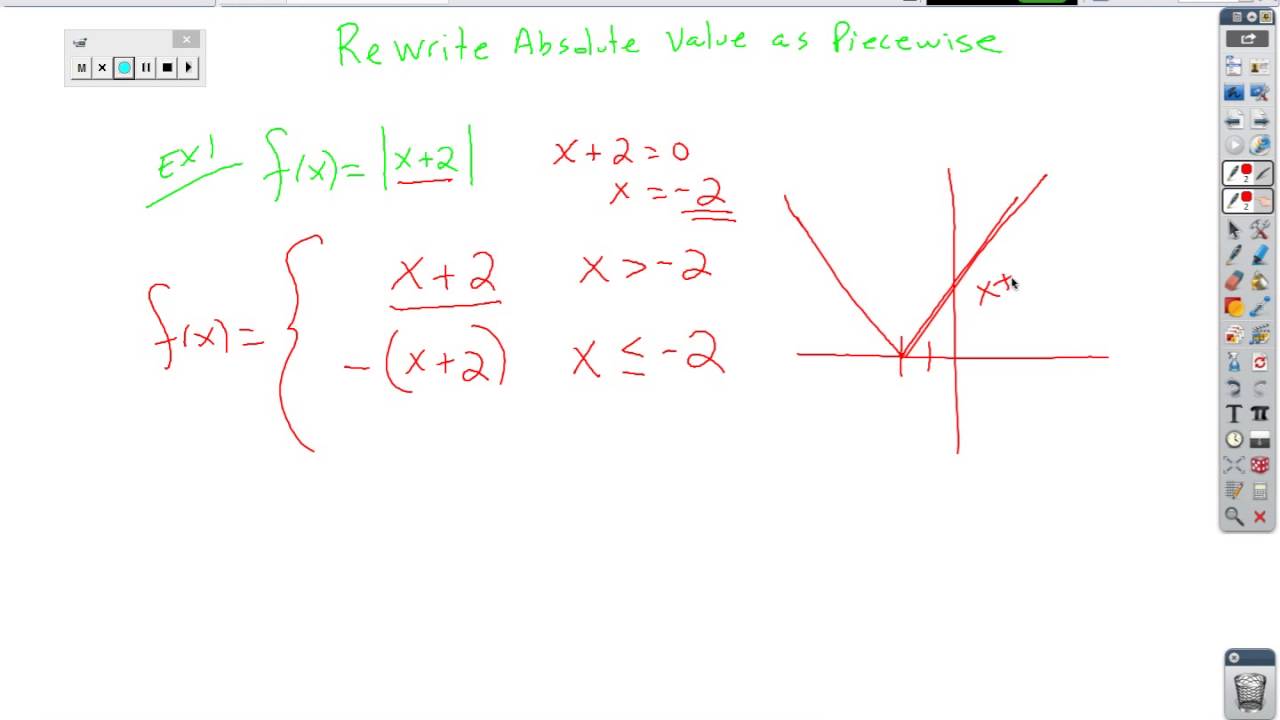
The absolute value of a function can be used to solve a variety of problems, such as finding the distance between two points, measuring the error in a calculation, and solving equations and inequalities. For example, the absolute value of the function $f(x) = x - 3$ is $|x - 3|$, which is equal to $x - 3$ if $x \ge 3$ and $-(x - 3) = 3 - x$ if $x < 3$. This information can be used to find the distance between two points on the graph of the function, or to solve the equation $|x - 3| = 2$.
The absolute value of a function is a fundamental concept in mathematics. It has a wide range of applications and is an essential tool for understanding many different types of problems.
Properties
These properties are important because they provide a deeper understanding of the behavior of the absolute value function. They also highlight the unique characteristics of the absolute value function that distinguish it from other functions.
- Non-negativity: The absolute value of a function is always non-negative because it is the distance between the function's output and zero. Distance is always non-negative, so the absolute value of a function is always non-negative.
- Symmetry: The absolute value of a function is symmetric about the y-axis because it is an even function. This means that the graph of the absolute value function is symmetric about the y-axis.
- Continuity: The absolute value of a function is continuous because it is a smooth function. This means that the graph of the absolute value function does not have any sharp corners or breaks.
These properties are essential for understanding the behavior of the absolute value function. They also provide a foundation for using the absolute value function to solve a variety of problems.
Applications
The absolute value of a function is a versatile tool that has a wide range of applications in mathematics. Some of the most common applications include:
- Finding the distance between two points: The absolute value of the difference between two numbers is equal to the distance between those two numbers on the number line. This can be used to find the distance between any two points in a coordinate plane.
- Measuring the error in a calculation: The absolute value of the difference between a calculated value and the true value is equal to the error in the calculation. This can be used to measure the accuracy of a calculation.
- Solving equations and inequalities: The absolute value of a function can be used to solve equations and inequalities. For example, the equation |x| = 3 can be solved by finding the values of x that make the absolute value of x equal to 3.
These are just a few of the many applications of the absolute value of a function. This versatile tool is an essential part of many different areas of mathematics.
Historical Context
The concept of the absolute value of a function is a fundamental one in mathematics. It has a wide range of applications, from finding the distance between two points to solving equations and inequalities. The absolute value of a function is defined as the distance between the function's output and zero. This means that the absolute value of a function is always non-negative.
The concept of the absolute value of a function was first developed by Ren Descartes in the 17th century. Descartes was a French mathematician and philosopher who is considered one of the founders of modern mathematics. He developed the concept of the absolute value of a function as a way to solve problems in geometry. Descartes' work on the absolute value of a function was published in his book La Gomtrie, which was published in 1637.
The absolute value of a function is a powerful tool that has a wide range of applications. It is an essential concept in mathematics, and it is used in many different fields, including physics, engineering, and economics.
Related Concepts
The absolute value of a function is closely related to the concepts of modulus and distance. The modulus of a complex number is its distance from the origin in the complex plane. The distance between two points in a coordinate plane is the absolute value of the difference between their coordinates. These concepts are all related because they involve measuring the distance between two points.
The absolute value of a function can be used to find the distance between two points on the graph of the function. For example, the absolute value of the function $f(x) = x - 3$ is $|x - 3|$, which is equal to $x - 3$ if $x \ge 3$ and $-(x - 3) = 3 - x$ if $x < 3$. This information can be used to find the distance between two points on the graph of the function, such as the distance between the points $(2, 1)$ and $(5, 4)$.
The absolute value of a function is also related to the concept of distance in a more general sense. The absolute value of the difference between two functions is a measure of how far apart the two functions are. This can be used to compare the accuracy of two different models or to measure the error in a calculation.
The absolute value of a function is a powerful tool that can be used to solve a variety of problems. It is an essential concept in mathematics, and it has a wide range of applications in many different fields.
Examples
The absolute value of a function is a fundamental concept in mathematics. It has a wide range of applications, from finding the distance between two points to solving equations and inequalities. One common example of the absolute value of a function is the function f(x) = x, which is equal to x if x is positive and -x if x is negative. This function is often used to represent the distance between a point and the origin.
- Finding the distance between two points: The absolute value of the difference between two numbers is equal to the distance between those two numbers on the number line. This can be used to find the distance between any two points in a coordinate plane.
- Measuring the error in a calculation: The absolute value of the difference between a calculated value and the true value is equal to the error in the calculation. This can be used to measure the accuracy of a calculation.
- Solving equations and inequalities: The absolute value of a function can be used to solve equations and inequalities. For example, the equation |x| = 3 can be solved by finding the values of x that make the absolute value of x equal to 3.
These are just a few of the many applications of the absolute value of a function. This versatile tool is an essential part of many different areas of mathematics.
Importance
The absolute value of a function is a powerful tool that has a wide range of applications in mathematics. It is used to find the distance between two points, measure the error in a calculation, and solve equations and inequalities. Without the absolute value function, many of these problems would be much more difficult or even impossible to solve.
- Finding the distance between two points: The absolute value of the difference between two numbers is equal to the distance between those two numbers on the number line. This is a fundamental concept in geometry and is used to solve a variety of problems, such as finding the length of a line segment or the area of a triangle.
- Measuring the error in a calculation: The absolute value of the difference between a calculated value and the true value is equal to the error in the calculation. This is important for measuring the accuracy of a calculation and for determining whether a calculation is within an acceptable margin of error.
- Solving equations and inequalities: The absolute value function can be used to solve equations and inequalities. For example, the equation |x| = 3 can be solved by finding the values of x that make the absolute value of x equal to 3. This technique can be used to solve a variety of equations and inequalities, including those that are difficult or impossible to solve using other methods.
These are just a few of the many applications of the absolute value function. It is a versatile tool that is essential for understanding many different types of mathematical problems.
FAQs - Absolute Value of a Function
This section provides answers to frequently asked questions about the absolute value of a function.
Question 1: What is the absolute value of a function?
The absolute value of a function is a measure of the distance between the function's output and zero. It is defined as the output of the function if the output is positive, or the negative of the output if the output is negative.
Question 2: What are the properties of the absolute value of a function?
The absolute value of a function is always non-negative, symmetric about the y-axis, and continuous.
Question 3: What are the applications of the absolute value of a function?
The absolute value of a function can be used to find the distance between two points, measure the error in a calculation, and solve equations and inequalities.
Question 4: What is the historical context of the absolute value of a function?
The concept of the absolute value of a function was first developed by Ren Descartes in the 17th century.
Question 5: What are the related concepts to the absolute value of a function?
The absolute value of a function is related to the concepts of modulus and distance.
Question 6: What is the importance of the absolute value of a function?
The absolute value of a function is a fundamental tool for understanding many different types of mathematical problems.
We hope this section has answered some of your questions about the absolute value of a function. If you have any further questions, please feel free to contact us.
Next Article Section: The Derivative of a Function
Conclusion
The absolute value of a function is a fundamental concept in mathematics. It has a wide range of applications, from finding the distance between two points to solving equations and inequalities. The absolute value of a function is always non-negative, symmetric about the y-axis, and continuous.
The absolute value of a function is a powerful tool that can be used to solve a variety of problems. It is an essential concept in mathematics, and it has a wide range of applications in many different fields.
The Ultimate Guide To Creating Keyspaces In Cassandra
Discover The Meaning Behind "Bunty": Unveiling The Shortened Form
Discover The Secrets: Do You Sand Between Coats Of Varnish?

Absolute Value Worksheet Pdf

Math Example Absolute Value Functions Example 2 Media4Math
crazyreti Blog